Time Remembered

November 22, 2015

One of the fundamental assumptions in physics, based largely upon mathematical concepts, is that time is reversible, that sequential activities can go “both ways,” an idea that led to many fanciful views of our “world.” Early writers of science fiction, H. G. Wells, for example, who in 1895 published “The Time Machine,” set the imaginations of countless humans on journeys fore and aft in time—and space. That this story anticipated work published by Einstein in 1905-6 is, at least, interesting.
Theoretical physics of today relies upon mathematical pondering; essays by physicists, in popular journals project a view of mathematics which I take to be a kind of religious honoring of a process which is, in the minds of some—including me—controversial. The controversy lies in the understanding of just what mathematics “is.” Is it a tool invented by Homo sapiens (faber) or is it something discovered, something that exists “out there” somewhere? See also the post-script to this essay.
Linguist and philosopher George Lakoff presented mathematics as a metaphorical expression of “embodied” mind, applying common understandings of motion, size, distance and so forth to the bodily extents. His significant book: “Where Mathematics Comes From: How the Embodied Mind Brings Mathematics into Being,” was published in 2000 with coauthor Rafael E. Núñez, a psychologist.1 My understanding of the book leads me to suspect that Lakoff has touched the nut of the matter. As theoretical physics lurches into metaphysical speculations about multiple universes, ‘branes,’ vibrating strings and super-symmetry, a population of doubters is arising and is generating cogent reactions, to present day theoretical physics, to what several writers have compared to “religious” fundamentalism. Indeed, this reverence for the “god” of mathematics can be found in samples from popular articles: “Finally, and perhaps most important, the discovery of the Higgs particle is an astonishing triumph of mathematics’ power to reveal the workings of the universe,” and the words of Nobel laureate Steven Weinberg: “Our mistake is not that we take our theories too seriously, but we do not take them seriously enough. It is always hard to realize that these numbers and equations we play with at our desks have something to do with the real world.”2
Those words from an article in Smithsonian by Brian Greene, only touch upon the reliance theoretical physics places upon mathematics. In a similar vein, there exists a kind of awe around persons of mathematical bent, as if they possess something mere mortals do not and therefore they deserve special attention. A recent book by mathematician Edward Frenkel, “Love and Math: The Heart of Hidden Reality,”3 was reviewed positively in the New York Review of Books (Dec. 5, 2013). A photograph of the author teaching in Berkeley caused me to reflect upon a short book by Erwin Schrodinger titled: Mind and Matter.4 Schrodinger writes: “So we are faced with the following remarkable situation. While the stuff from which our world picture is built is yielded exclusively from the sense organs as organs of the mind, so that every man’s world picture is and always remains a construct of his mind and cannot be proved to have any other existence, yet the conscious mind itself remains a stranger within that construct, it has no living space in it, you can spot it nowhere in space.” Further along: “The reason why our sentient, percipient and thinking ego is met nowhere within our scientific world picture can easily be indicated in seven words: because it is itself that world picture. It is identical with the whole and therefore cannot be contained in it as a part of it. But, of course, here we knock against the arithmetical paradox; there appears to be a great multitude of these conscious egos, the world however is only one. This comes from the fashion in which the world-concept produces itself.”
Schrodinger also ponders the idea, attributed to Sherrington, that the “world” (our world, our earth ) existed for billions of years before a brain evolved to contemplate it. Carl Sagan, always the startling observer, says it this way: “We are a way for the cosmos to know itself.” Sagan, a man who knew himself well enough continues: “But I could be wrong.”
I wonder how often that phrase (“but, I could be wrong”) enters the thoughts of the several generations of physicists who have hung their hopes and written their theses and several thousand papers about supersymmetry and string theory? In view of the awakening happening in some of us, could this portend another paradigm shift? We shall see.
In the spirit of Schrodinger, whose translator has made difficult the grasp of the essence of his meaning, I will attempt my own paraphrase: Notice that the mind which is the source of the image of our world appears no-where in that image. Why should that be? Mathematics at base is (or seems to me) a grand tautology, an enormous rule book, perhaps a version of the “Glass Bead Game,” of Herman Hesse.5 It is significant that Hesse’s solution to the problem faced by the Magister Ludi—the Master of the Game—was to have the master leave the game and re-enter “life.”
I am not proposing that mathematics is not useful nor that we abandon applied mathematics—nor even abandon conceptual or pure mathematics. I ask only that we attempt to see ourselves doing what we are doing— as Schrodinger has done.
In regard to the “Higgs,” of which Greene was speaking when he wrote that essay, has it ever entered the mind of anyone but myself that almost the very nature of the ‘experiment,’ the LHC, the fantastic costs, the astounding computational power required, the large numbers of also rans and finally the small number of individuals who, when they encounter a spot on a viewing screen, somehow “see” that spot as a Higgs event? How can we come to understand the coding involved in the computer programs involved? To this miniscule number of humans (in comparison to the 7 Billion others) a significant portion of a lifetime would be required to even suspect that an “event” was happening. And, in the spirit of the scientific method, how is this “event’” to be verified independently? Do we manufacture several other LHC’s in other countries, organized by other humans with independent status, to verify this? No, that will not happen. So, we are left with a published “event,” whose importance, in respect of the costs involved, raises the question of “reality.” The outcome almost demands positive support. After all, can you imagine that someone will publish, after spending years of his life and the supporters, tens of billions of dollars, “We sought the Higgs, but did not find it.”
No, there are no such journals, no honesty of this kind. All journal articles in my experience run to the same form: we thought about this idea, we searched the literature, we got a grant, and “ta da,” we were right. How rare, if it exists at all, is the form: “. . . “ta da,” we were wrong.”
Also, in the words of physicist John Horgan, ”The Higgs doesn’t take us any closer to a unified theory than climbing a tree would take me to the moon.”
Let me try to show this mathematical and Schrodinger problem in pictographic form: Here is an imaginary picture of a Physicist (his brain) imagining a formula:

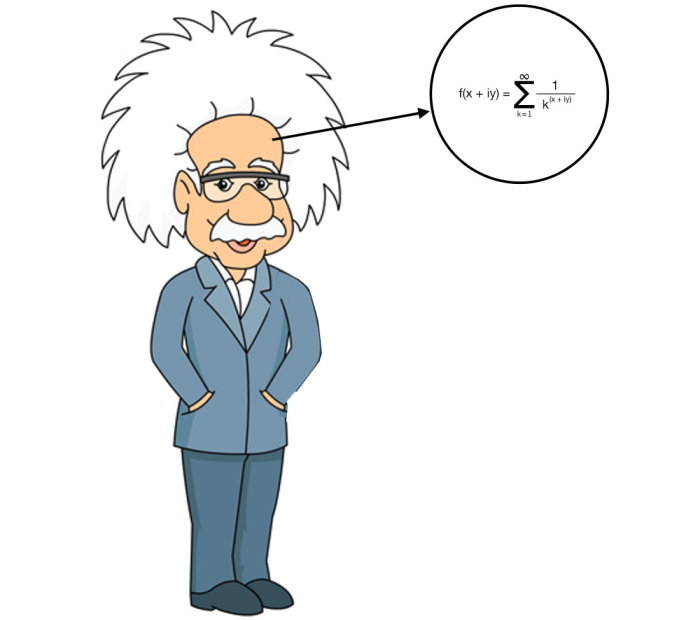
Here is another imaginary picture of the Physicist teaching about the formula. Notice that he is pointing to his head, which contains his brain, the brain that imagined the formula.

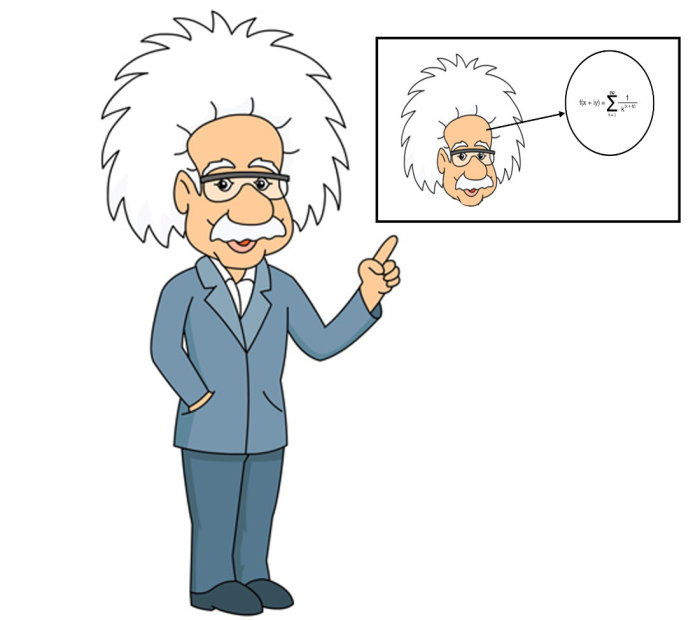
However, in the Physicist’s world, and in the world described by Schrodinger, here is what he is teaching, and this is what is purported to be the world—”out there:”

Magically, the brain of the human who created the world, “out there,” is no longer part of the world “out there.” I wonder what happened to it? Well, “out there” surely reflects back upon this brain, my brain, in the form of: let us now return to time. Because the brain of a human can imagine a tautologically consistent idea such as time reversal, the idea returns as if it were a spirit from the world “out there,” and forces us to further imagine—because we are now faced by a form of reality from which is absent the cause of that reality—other ideas, among which are multiple universes, super-symmetrical existences, strings, “branes,” and so forth. Have we returned to the land of Alice, the invention of Charles Lutwidge Dodgson, not so ironically a mathematician? Is there anything in the world of reality that even suggests that time is reversible—is there a mirror image of itself, like a symmetry in physics or mathematics? The physicists claim that there are three symmetries: time, charge and space. Yet nowhere has anyone witnessed time reversed—although with a motion camera, we can make it appear to happen. Film me while I drop an egg on the floor to make a mess; run the film backwards and the egg re-assembles itself and leaps into my hand. Will that happen in the world of ‘reality,’ the world of the theoretical physicist who claims it should do just that? Parity, which is what the laws of physics demand conserving, has been shown to lack conservation in several areas: the disintegration of an isotope of cobalt decays asymmetrically, optical isomers are sometimes not symmetrical. In short our theoretical picture of the physical world is imperfect—and by extension, therefore—so must be our mathematical conception of the physical world. I continue to remind that it is “us” (ourselves) we are employing when we employ mathematics (as so importantly demonstrated by Lakoff and Nunez). This matter of time and its relation to reality has recently been opened to discussion by theoretical physicist, Lee Smolin. His book: “Time Reborn,”6 has been described as “The strongest dose of clarity in written form to have come along in decades. The implications go far beyond physics, to economics, politics, and personal philosophy. Time Reborn places reality above theory in stronger and clearer terms than ever before, . . .” Well, perhaps it is a strong dose of clarity. Coming just about 100 years beyond Einstein’s conjunction of time and space, Smolin suggests their separation is necessary for us to move forward in our ideas about reality. Who but an individual separated from reality could believe-in (and these are significant words—for modern theoretical physics resembles a belief) time reversal, time symmetry, again necessary for the ideas of modern physical theories to be consistent. As I was pondering these ideas, I recently read a perspective review in the journal “Science,” (December 6, 2013) which described recent investigations of individual mammalian cells. Most experimentation in the past has been based upon substrates obtained by separating the contents of cells into mixtures, with experimental work done using those mixtures to show us a picture of what the cell is doing. Just how wrong we may have been about cellular activities by using this method has been recently shown by a variety of investigators and reviewed by Levine etal in Science, 342-1193, 2013. By methods that include time-lapse photography, investigators are able to show that key regulatory functions, in individual cells, pulse on and off, in a pattern specific to a diverse variety of cells from bacteria through mammalian cells. These pulsations regulate the genetic engines of the cells, processes masked by earlier methods. In short, an individual cell, from microbes to mammals thus suggesting commonality of behavior, has some form of internal “clock,” (my word) one that infers time and also infers a unidirectional vector dimension to time. This kind of information rests upon empirical data, reproducible amongst other laboratories, unlike the world of theoretical physics, as I have tried to show above. Its implications are equally startling and can evoke mental wool gathering, but, in this case, based upon data that is potentially reproducible and knowable to any student with the proper interest. If individual cells possess this repetitive pulsatile behavior relative to important intracellular (cellular) activities, perhaps the organism as a whole, if, unlike microbes, are joined together in the form of organs and tissues, some of our observed temporal organic activity originates within individual cells. I often think of my own pulsatile behavior, my tendency to enthuse for a while over some project only to find myself moved in another direction within a period of time (there’s that word again). And, I am enthused by the reports and observations of some of our present-day thinkers. I suspect that theoretical physics has devolved into a system of beliefs; contrary speculation, even empirical data, is not welcomed. Yes, I do want the ‘pure’ mathematicians to continue their probing, for what they are doing is probing the limits of the human brain, and that is a good thing. Let us caution, however, that they not take their journeys beyond the human brain and come to believe they have found something that exists apart from us—something “out there.” Imaginary worlds are fun for many; they make entire lives for some of us: priests and popes and mullahs. They are also the root cause of most of humanity’s strife and stress. As the lyricist, Oscar Hammerstein reminds us (In “South Pacific”) “You’ve got to be carefully taught to hate and fear, before it’s too late, before you are six, or seven, or eight.” Perhaps along with usual courses in mathematics, mathematicians should also learn where what they are doing comes from.

1. George Lakoff and Rafael Núñez, 2000, Where Mathematics Comes From. Basic Books. ISBN 0-465-03770-4

2. The First Three Minutes: AModern view of the Origin of the Universe, Steven Weinberg, Basic Books, 1977, ISBN 13: 9780465024353

3. E. Frenkel: “Love & Math, The Heart of Hidden Reality”, Basic Books, 2013, ISBN-13: 978-0465064953.

4. Erwin Schrodinger, “Mind and Matter,” Cambridge University Press, 1958, ASIN: B0028L2CC8.

5. Herman Hesse, “The Glass Bead Game (Magister Ludi),” Henry Holt and Co, 1943.

6. Lee Smolin, “Time Reborn: From the Crisis in Physics to the Future of the Universe,” Houghton,Mifflin, Harcourt, 2013, ISBN 978-0547511726.

Post Script: There appeared in the New York Review of Books (Dec. 3, 2015 issue) a review of a rmarkable book by Michael Harris. The review concedes that mathematics is indeed a construction of mankind and the author of the book goes further: “. . . pure mathematics must concede the futility of its own quest for ultimate truth. As Harris observes, “every veil lifted only reveals another veil.” The mathematician is doomed to what André Weil called an endless cycle of “knowledge and indifference.” Michael Harris “Mathematics Without Apologies: Portrait of a Problematic Vocation”Michael Harris Princeton University Press, 2015, ISBN-13: 978-0691154237

Reviewed: http://www.nybooks.com/articles/archives/2015/dec/03/mountains-mathematics/

(c) copyright J.C. Leissring 2015